Part a

Laboratory Math

This is Part A, Laboratory Math, under the module topic, Measurements, Solutions, Dilutions. This topic part has one section: Content Tutorial.

Content Tutorial

Laboratory Math

Review the procedures below that you will need to know in order to perform basic mathematical calculations in the laboratory. You may check your knowledge by doing the practice problems at the end of each part of this section.

Part 1: Metric System

This is the numerical language of science. Base units that you will most often use in this class are meters, grams, liters, and moles. These units will be appended with prefixes to modify the unit by a power of ten.

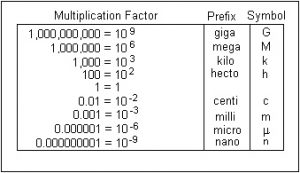
Practice problems:

1. The distance between two cells in 800 µm. How many mm is that?
2. The amount of sorbitol you want to weigh is 1.9 g. How many mg is that?
3. The volume you want to measure is 100 ml. How many liters is that?
4. Your reaction generates 0.1 µmoles of product. How many mmoles is that?

Scientific notation expresses numbers so there is one digit to the left of the decimal point and that number is multiplied by a power of ten. 2334 becomes 2.334 x 10³ and 0.0041 becomes 4.1 x 10^-3. Computations are easier with numbers in scientific notation and some numbers that are easier to write (602,214,199,000,000,000,000,000 versus 6.02 x 10²³).

Practice problems:

Convert the following to scientific notation

1. 1000
2. 2
3. 0.0023
4. 0.000000467

The metric system and scientific notation go hand in hand, making unit conversions straightforward. For example 100 µl can be converted to ml by writing the starting volume in scientific notation (1.00 x 10² µl) and multiplying by the power of ten that separates the units (1 ml = 1 x 10³ µl). Set up every equation so the units will cancel properly when you multiply through.

Practice problems:

Be sure you can express your answers in scientific notation.

1. How many ml is 100 µl?
2. How many mg is .023 g?
3. How many mmoles is 250 µmoles?

Part 2: Concentrations

Molarity (moles/liter) is a common expression of concentration. When making a solution of a particular molarity, you need to know three things: the desired molarity, the desired volume and the formula weight of the compound to be dissolved. The best place to find the formula weight (grams/mole) is on the chemical’s bottle. Calculations are performed by setting up an equation so that the units cancel, leaving grams in the numerator and volume in the denominator.

Another common expression of concentration is percent. Percent solutions are always based on 100 ml. For powdered substances, percent solutions reflect the weight in a 100 ml volume (“w/v”). For example a 10% solution of NaCl is 10 grams in 100 ml of water. In fact a 10% solution of any powdery substance is 10 grams in 100 ml. For liquids, percent solutions reflect the volume in a 100 ml final volume (“v/v”). For example a 70% ethanol solution is 70 ml of 100% ethanol and 30 ml of water. Remembering that 1 ml of water weighs 1 gram may help you remember the w/v and v/v expressions.

Practice problems:

1. You want to make 100 ml of a 0.5M sorbitol solution. The formula weight of the substance you want to dissolve is 182. How many grams will you measure?
2. You want to make 10 ml of a 0.01% (w/v) solution of XC. How many grams will you dissolve?
3. How would you make 100 ml of a solution that is 5% (v/v) acetic acid and 5% methanol?

Part 3: Dilutions

Many solutions are made by diluting concentrated stock solutions. Dilution factors of 1:2, 1:5, 1:10 and 1:100 are common. These dilutions are made by diluting one “part” stock with 1, 4, 9 or 99 “parts” water. For example, you could make 100 ml of a 0.5M sorbitol solution by mixing 10 ml of a 5M stock solution with 90 ml of water. This is a 1:10 dilution of the stock. The dilution factor can be converted to a fraction to determine the solution’s final concentration (5M x 1/10 = 0.5M).

When the dilution factor is less obvious, the formula C₁V₁ = C₂V₂ can be used, where C₁ is the starting concentration of the stock solution, C₂ is the desired concentration, V₁ is the volume of stock you’ll need (usually this is your unknown) and V₂ is the final volume you want to make. For example, to make 1000 ml of a 0.2M Tris from a 1.5M stock you would multiply 1.5M (V₁) = 0.2M (1000) to find that you will need 133 ml of the stock. To determine how much water to add you would subtract V₂ – V ₁ , in this case 1000 ml –133 ml = 867 ml of water.

When solutions must be diluted several orders of magnitude, then serial dilutions are made. The concentrated stock is progressively diluted, for example using a 1:100 dilution as the new “stock” in another 1:100 dilution. Such a serial dilution produces a solution that is 10,000 times less concentrated than the starting material. One benefit to serial dilutions is that small volumes of each dilution can be made accurately. A drawback is that any pipetting or calculation error is propagated through every dilution.

Part b

Solutions & Dilutions

This is Part B, Solutions and Dilutions, under the module topic Measurements, Solutions, and Calculations. This topic part has two sections: Content Tutorial and Animations.

Content Tutorial

Solutions & Dilutions

Solutions & Dilutions Tutorials: (Mixtures and Solutions; Water and Glassware for Solution Making; Volumes, Amounts, and Concentrations; Formulas for Solutions; Examples: Making Solutions; Making Dilutions; Working with Stock Solutions) *Begin with Mixtures and Solutions tutorial and continue on to the following sections by clicking on the purple arrow on the right-hand side of the screen.

• Mixtures Tutorial (www.ruf.rice.edu, Multimedia Page)
• Dilutions Tutorial (abacus.bates.edu, Multimedia Page)

Animations

Review the following animations on Chemical Mixtures, Solutions, and Dilutions from the Baylor College of Medicine to familiarize yourself with the techniques and procedures for working with liquids, gases, and solids in a molecular biology laboratory.

BioEd Online: Baylor College of Medicine – Online Presentations

a. Quantitative Methods: Solutions & Dilutions” by David R. Caprette, Ph.d. Rice University Department of Biochemistry & Cell Biology(www.bioedonline.org, Multimedia Page)

b. Quantitative Methods: Diluting Solutions Part 2” by David R. Caprette, Ph.d. Rice University Department of Biochemistry & Cell Biology(www.bioedonline.org, Multimedia Page)

c. An Introduction to Chemical Mixtures” by David R. Caprette, Ph.d. Rice University Department of Biochemistry & Cell Biology (www.bioedonline.org, Multimedia Page)